Why are Gas Laws Important in Chemistry? Gas Laws Chemistry
Gas laws are fundamental principles of chemistry that describe the behavior of gases under different conditions. These laws explain how gases behave in terms of pressure, volume, temperature, and the number of gas particles present.
Understanding gas laws is important in various fields, including chemistry, physics, and engineering. In this article, we will discuss the four primary gas laws: Boyle’s law, Charles’s law, Gay-Lussac’s law, and the combined gas law.
Boyle’s Law
Boyle’s law states that at a constant temperature, the pressure of a gas is inversely proportional to its volume. In other words, as the volume of a gas decreases, its pressure increases, and as the volume increases, its pressure decreases.
Boyle presented this law in 1662.
According to this law: –
At a constant temperature, the volume (V) of a fixed quantity of a gas is inversely proportional to its pressure (P).
V ∝ 1/P
V = k x 1/P (where k is a constant)
PV = k
Therefore, the product of pressure and volume of a given amount of gas at a constant temperature is always constant.
If the volume of a certain amount of a gas at the same temperature is V1 and P1 and its volume is changed from V1 to V2, then its pressure from P1 to P2 will be as follows
P1V1 = P2V2
- Importance of Biomolecules in Life || What are the 4 main biomolecules?
- Resonance effect or mesomeric effect || What is resonance effect with example?
- Glucose Structure: Physical and chemical properties, Glucose Chemical Reaction
- Valency of Elements || How to Find Valency || What is the Valency of the atom?
- Introduction of Inductive-Effect || How does Inductive Effect Work?
- IUPAC Name : How to find the IUPAC name of compounds.
This law can be expressed mathematically as P1V1 = P2V2, where P1 and V1 are the initial pressure and volume, and P2 and V2 are the final pressure and volume, respectively.
Boyle’s law is commonly observed in everyday life, such as when inflating a bicycle tire. When the pump is attached to the tire valve, the volume of the air inside the tire decreases, causing the pressure to increase until it reaches the desired level.
Charles’s Law
Charles’s law states that at a constant pressure, the volume of a gas is directly proportional to its temperature in kelvin. In other words, as the temperature of a gas increases, its volume increases, and as the temperature decreases, its volume decreases.
This law can be expressed mathematically as V1/T1 = V2/T2, where V1 and T1 are the initial volume and temperature, and V2 and T2 are the final volume and temperature, respectively.
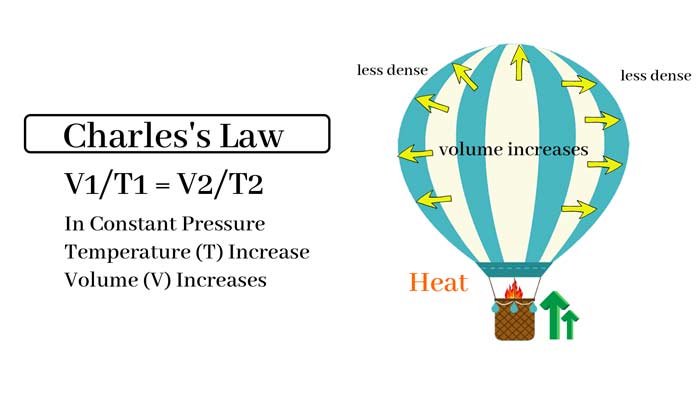
An example of Charles’s law in action is a hot air balloon. As the air inside the balloon is heated, its volume increases, causing it to become less dense than the surrounding air. This buoyancy causes the balloon to rise.
Gay-Lussac’s Law
Gay-Lussac’s law states that at a constant volume, the pressure of a gas is directly proportional to its temperature in kelvin. In other words, as the temperature of a gas increases, its pressure increases, and as the temperature decreases, its pressure decreases.
- Importance of Biomolecules in Life || What are the 4 main biomolecules?
- Resonance effect or mesomeric effect || What is resonance effect with example?
- Glucose Structure: Physical and chemical properties, Glucose Chemical Reaction
- Valency of Elements || How to Find Valency || What is the Valency of the atom?
- Introduction of Inductive-Effect || How does Inductive Effect Work?
- IUPAC Name : How to find the IUPAC name of compounds.
This law can be expressed mathematically as P1/T1 = P2/T2, where P1 and T1 are the initial pressure and temperature, and P2 and T2 are the final pressure and temperature, respectively.
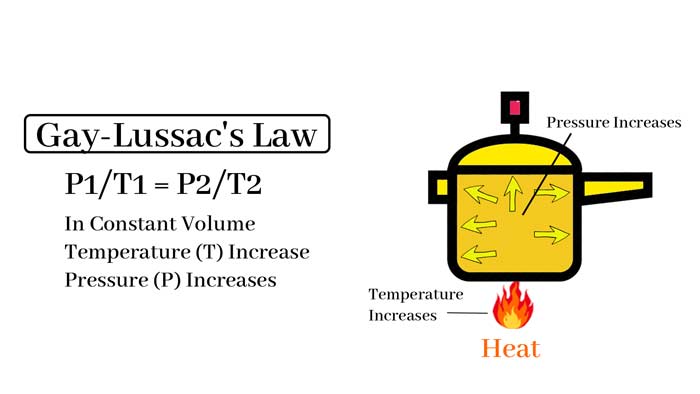
One practical application of Gay-Lussac’s law is in the operation of a pressure cooker. As the temperature inside the cooker increases, the pressure also increases, causing the food to cook faster than it would at normal atmospheric pressure.
Combined Gas Law
The combined gas law combines Boyle’s law, Charles’s law, and Gay-Lussac’s law into a single equation that relates the pressure, volume, and temperature of a gas. The equation is expressed as P1V1/T1 = P2V2/T2, where P1, V1, and T1 are the initial pressure, volume, and temperature, and P2, V2, and T2 are the final pressure, volume, and temperature, respectively.
Applications of the combined gas law can be seen in many real-world scenarios, such as in the compression and storage of natural gas.
Conclusion
In conclusion, gas laws are fundamental principles of chemistry that describe the behavior of gases under different conditions. Understanding these laws is important in various fields, including chemistry, physics, and engineering.
The four primary gas laws – Boyle’s law, Charles’s law, Gay-Lussac’s law, and the combined gas law – explain how gases behave in terms of pressure, volume, temperature, and the number of gas particles present in Gas Volume.
