Solid State Notes: CBSE Class 12 Chemistry Notes and DPP

Solid State

Solid is a state of matter which has strong intermolecular forces of attraction.

The melting point of solids will be much above the room temperature. 

For more detail join us on Link to Join telegram group https://t.me/joinchat/NC9eVRt24PWWoVp… Link to Join telegram channel https://t.me/sanjaychemistrypage Registered your mail id here for the online test help@chemistrypage.in

NEET Exams 2021 Chemistry Notes The Solid State

Download Solid state short notes pdf file.

Download

The Solid State Practice Set with Solution

Try it for free. No registration needed. But don’t forget to follow us our Social Pages

Download

Some of the characteristic features of solids are

  1. Strong intermolecular forces and short intermolecular distances
  2. Rigid, possess definite shape, size and volume
  3. Solids are almost incompressible
  4. Solids have high density
  1. Solids have negligible fluidity
  2. The diffusion of the particles in the solid state is negligible

Types of Solids

Based on the three-dimensional arrangement of particles in space, the solids are classified into two types.

  • Crystalline solids
  • Amorphous solids
S. No.Crystalline solids
Amorphous solids
1Long range orderly arrangement of constituents.Short range, random arrangement of constituents.
2Definite shapeIrregular shape
3Generally crystalline solids are anisotropic in naturethey are isotropic * like liquids
4They are true solidsThey are considered as pseudo solids or super cooled liquids
5Definite Heat of fusionHeat of fusion is not definite
6They have sharp melting points.Gradually soften over a range of temperature and so can be moulded
7NaCl, Diamond etc.Rubber, Plastics, Glass etc.

Classification of Crystalline Solids

Based on the nature of inter-molecular forces and the component particles, crystalline solids are classified into four types

  1. Molecular Solids
  2. Ionic Solids
  3.  Metallic solid
  4. Covalent solid

Molecular Solids

  1. These solids have molecules as the component particles.
  2. In these crystals the molecules are held together by weak vander Waal’s forces of attraction.
  3. These are subdivided into three types. 
  • Non polar Molecular Solids
  • Polar Molecular Solids
  • Hydrogen Bonded Molecular Solids

Non polar Molecular Solids

They contain either atoms (Ar and He) or the molecules formed by non polar covalent bonds for example H2, Cl2, CH4, and I2

  • The atoms or molecules are held by weak dispersion forces or London forces
  • They are soft and non-conductors of electricity
  • They have low melting points and are usually in liquid or gaseous state at room temperature and pressure. 

Polar Molecular Solids

They contain substances like HCl, SO2 etc.

1)      They are held together by stronger dipole-dipole interactions.

2)      These are soft and non-conductors of electricity.

3)      Their melting points are higher than those of non polar molecular solids

4)      Solid SO2 and solid NH3are some examples of such solids. 

Hydrogen Bonded Molecular Solids

1)      They contain polar covalent bonds between H and F, O or N atoms.

2)      Strong hydrogen bonding binds molecules of such solids like H2O (ice). 

3)      They are non-conductors of electricity.

4)      Generally they are volatile liquids or soft solids under room temperature and pressure.

Ionic Solids

1)      They contain ions are the constituent particles

2)      The ions are held together by strong electrostatic force of attraction (Ionic bond).

3)      These are hard and brittle in nature.

4)      They possess high melting point.

5)      These are non-conductors in solid state but conduct in solution and molten state.

Ex: NaCl, KCl, NaNO3

Metallic solid

1)      In these solids metal ions known as Kernels are the constituent particles.

2)      These kernels are held together by a sea of freely moving electrons.

3)      These solids are good conductors of electricity in solid state as well as molten state due to the presence of freely moving electrons.

4)      These solids are malleable and ductile.

5)      They possess a lustrous nature due to the presence of free electrons.

Ex: All metals except mercury

Covalent solid

1)      These solids have non metal atoms as their constituent particles.

2)      These atoms are held together by strong covalent bonds.

3)      They are also called as giant molecules. 

4)      They are very hard and brittle.

5)      They possess very high melting point and may even decompose before melting.

Ex: Diamond, Graphite

Crystal Lattices and Unit Cells

A regular three dimensional arrangement of points in space is called a crystal lattice.

The following are the characteristics of a crystal lattice

1)      Each point in the lattice is called lattice point or lattice position (location).

2)      Each point in the crystal lattice represents one constituent particle which can be an atom, molecule or ion.

3)      The lattice point are connected by straight lines, so that the geometry of the lattice can be indicated.

Unit Cell

It is the smallest portion of the crystal lattice which when repeated in three dimensions generates the entire lattice.

Each unit cell is characterised by 6 basic parameters.

(a) Unit lengths (a, b and c) along three edges of the unit cell

(b) Three angles (α, β and γ) between the three edges. 

Types of Unit Cell

Primitive (Simple) Unit Cell

Face Centred Unit Cell

Body Centred Unit Cell

Packing in Crystal

Close Packed Structures

(a)    Close Packing in One Dimension

(b)    Close Packing in Two Dimensions

(c)    Close Packing in Three Dimensions

(a) Close Packing in One Dimension

  1. The number of nearest neighbors of a particle is called its coordination number.
  2. Thus, in one-dimensional close-packed arrangement, the coordination number is 2.

(b) Close Packing in Two Dimensions

Square close packing

Co-ordination number is 4

Hexagonal close packing

Co-ordination number is 6. 

(c) Close Packing in Three Dimensions

1. Square close-packed layers

The lattice thus generated is the simple cubic lattice, and its unit cell is the primitive cubic unit cell

2. Hexagonal close packed layers

Types of Voids

(Hexagonal close packed layers)

Size of octahedral and tetrahedral voids

If the R is the radius of the sphere in the close packed structure

Radius (r) of tetrahedral voids = 0.225 R

Radius (r) of octahedral voids = 0.414 R

In ionic solids, the bigger ions (usually anions) form the close packed structure and the smaller ions (usually cations) occupy the voids.

Radius of cation r+ occupy the tetrahedral voids = 0.225 r-

Radius of cation r+ occupy the octahedral voids = 0.414 r-

If the latter ion is small enough then tetrahedral voids are occupied, if bigger, then octahedral voids.

Not all octahedral or tetrahedral voids are occupied. 

Locating Tetrahedral and Octahedral Voids

We know that close packed structures have both tetrahedral and octahedral voids.

Let us take ccp (or fcc) structure and locate these voids in it. 

Locating Tetrahedral Voids

Let us consider a unit cell of ccp or fcc lattice

  1. There is one tetrahedral void in each small cube and eight tetrahedral voids in total.
  2. Each of the eight small cubes have one void in one unit cell of ccp structure.
  3. We know that ccp structure has 4 atoms per unit cell.
  4. Thus, the number of tetrahedral voids is twice the number of atoms. 

Locating Octahedral Voids

Let us consider a unit cell of ccp or fcc lattice

One octahedral void at the body centre of the cube.

One octahedral void at the centre of each of the 12 edges

Radius Ratio Rule

For the stability of an ionic compound each cation should be surrounded by maximum number of anion and vice versa

The umber of opposite charge ions surrounding each ion is called its coordination numbers

Radius (r) of tetrahedral void = 0.225 R;

Radius (r) of octahedral void = 0.414 R

where R is the radius of the spheres in close packing.

Greater the radius ratio the largest is the size of the cation and hence greater is its CN

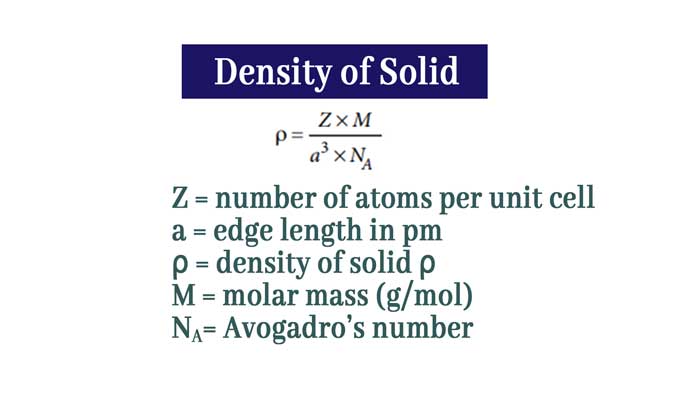
Calculations of density of Solids

Z = number of atoms per unit cell

a = edge length in pm

ρ = density of solid ρ

M = molar mass (g/mol)

NA= Avogadro’s number

Imperfections in solids

The defects are basically irregularities in the arrangement of constituent particles.

Point defects

The irregularities or deviations from ideal arrangement around a point or an atom in a crystalline substance

Line defects

The irregularities or deviations from ideal arrangement in entire rows of lattice points

Types of Point Defects

1) stoichiometric defects

  1. Vacancy defect
  2. Interstitial defect
  3. Schottky defect
  4. Frenkel defect

2) impurity defects

3) non-stoichiometric defects

  1. Metal excess defects
  2. Metal deficiency defect

1. Vacancy defect

  1. When some of the lattice sites are vacant
  2.  This results in decrease in density of the substance.
  3. This defect can also develop when a substance is heated.

 2. Interstitial defect

  1. When some constituent particles occupy an interstitial site
  2. This defect increases the density of the substance

Frenkel and Schottky Defect

  1. Vacancy defect in ionic solid
  2. Electrical neutrality maintain
  3. AgBr shows both, frenkel as well as schottky defects

Impurity Defects

  1. If molten NaCl containing a little amount of SrCl2 is crystallised
  2. Some of the sites of Na+ ions are occupied by Sr2+
  3. Each Sr2+ replaces two Na+ ions.
  4. It occupies the site of one ion and the other site remains vacant.
  5. The cationic vacancies thus produced are equal in number to that of Sr2+ ions.
  6. Example SrCl2, CdCl2 and AgCl.

3) Non-Stoichiometric defects

1. Metal excess defects

2. Metal deficiency defect

1. Metal excess defects

Metal excess defect due to anionic vacancies

  1. This happens by loss of electron by sodium atoms to form Na+ ions.
  2. The released electrons diffuse into the crystal and occupy anionic sites
  3. As a result the crystal now has an excess of sodium.
  4. The anionic sites occupied by unpaired electrons are called F-centres.
  5. They impart yellow colour to the crystals of NaCl.
  6. The colour results by excitation of these electrons when they absorb energy from the visible light falling on the crystals.
  7. Similarly, excess of lithium makes LiCl crystals pink and excess of potassium makes KCl crystals violet

1. Metal excess defects

Metal excess defect due to the presence of extra cations at interstitial sites

  1. Zinc oxide is white in colour at room temperature.
  2. On heating it loses oxygen and turns yellow.

 

  1. The excess Zn2+ ions move to interstitial sites and the electrons to neighbouring interstitial sites.
  2. Now there is excess of zinc in the crystal and its formula becomes Zn1+XO.

 (ii) Metal Deficiency Defect

  1. In metal deficiency defect, a cation is missing from its lattice site.
  2. To maintain electrical neutrality, one of the nearest metal ions acquires an extra positive charge.
  3. This type of defect occurs in compounds where the metal can exhibit variable valency. e.g., Transition metal compounds.
  4.  Example: Ferrous oxide, Nickel oxide.

 Conduction of Electricity in Semiconductors

  1. Their conductivity is increased by adding an appropriate amount of suitable impurity.
  2. This process is called doping.

 Doping

By adding an appropriate amount of suitable impurity to increase the conductivity of semiconductors.

 Applications of n-type and p-type semiconductors

  1. They are used for making electronic components.
  2. Diode is a combination of n-type and p-type semiconductors and is used as a rectifier.
  3. Transistors are type of semiconductor used to detect or amplify radio or audio signals.
  4. The solar cell is an efficient photo-diode
Tags: , , , , ,

Leave a Reply