Electric Cell : E.M.F., Terminal Potential, Internal Resistance
An electric cell is a device that converts chemical energy into electrical energy and maintains a constant flow of charge in a circuit. It consists of two rods of different metals.
Which are called electrodes or plates. It is immersed in a liquid called electrolyte. This liquid has this property that when the plates are immersed in it, one plate becomes positively charged and the other becomes negatively charged.
When both the plates are connected with a wire, charge starts flowing in the wire. Such a chemical reaction takes place in the electrolyte inside the cell, due to which the charges on the plates are supplied and the charge flows in the wire.
In this way the cell keeps on converting chemical energy into electrical energy.
Electromotive force (EMF) of a Cell
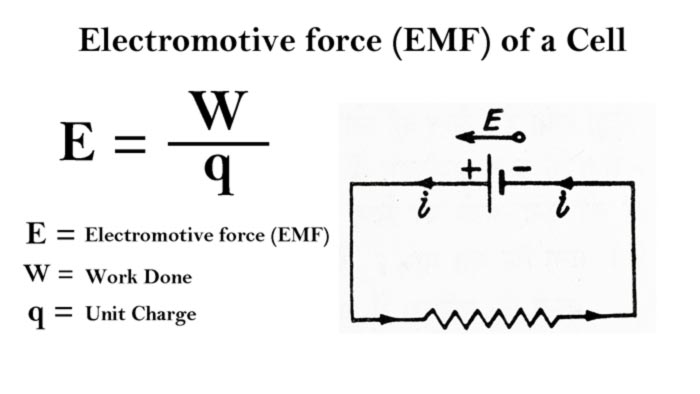
To maintain the continuous flow of charge in an electric circuit, some work has to be done. This work is done by the cell. The energy that is liberated in the chemical reactions taking place in the cell is what drives the charge in the circuit.
In this way the cell converts the chemical energy of its electrodes and electrolytes into electrical energy.
The energy given by the cell in passing the unit charge through the whole circuit is called Electromotive force (E. M. F.). E.M.F. is a characteristic of each cell, which depends on the nature of the plates and electrolytes used in the cell. It is not affected by the amount of electrolyte and the size of the plates or the distance between them.
If W is the energy given by the cell in passing a q coulomb charge in a circuit, then the EMF of the cell:
E = W/q Joule/coulomb
The unit of EMF is joule/coulamb which is called Volt. If the energy given by the cell on passing a charge of 1 coulomb in a circuit is 1 joul, then the EMF of the cell is 1 Volt.
As is clear from the figure, the direction of the current sent by the cell in the circuit is from the negative electrode to the positive electrode ‘within the cell’.
- Mole in chemistry: What is it and how to calculate it?
- What are the common uses of cesium in industry : Properties, Isotopes
- What is aluminum bromide and how is it used in industry?
- Exploring the Rankine Scale: Understanding the Basics and Applications
- What is Empirical Formula? How to Calculate Empirical Formula?
In other words, the positive charge inside the cell flows from a lower potential to a higher potential. Therefore, the EMF of the cell is directed from the negative electrode to the positive electrode inside the cell.
Terminal Potential Difference
Let the EMF(E) of a cell connected to an electric circuit and W is the energy given by the cell when q charge flows through the circuit. Then the charge q will be the same in all parts of the circuit. If W1, W2, W3, …. are the energy expended in different parts of the circuit, then their sum will always be W.
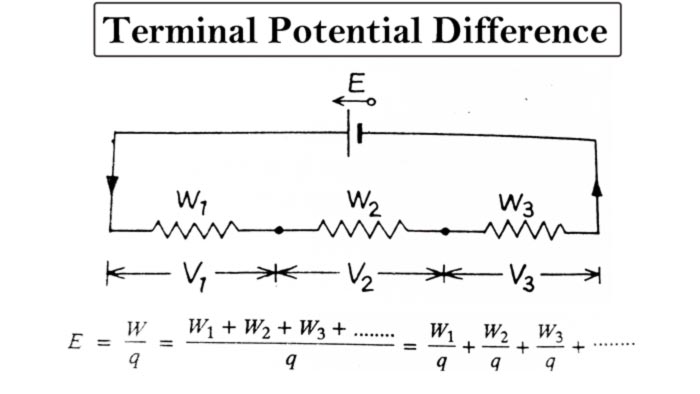
Therefore
Let W1 / q = V1, W2 / q = V2, W3 / q = V3 ……then
E = V1 + V2 + V3 + ….
It is clear that V1, V2, V3, … is the energy spent in different parts of the circuit in carrying a unit charge. These are called terminal potential differences of different parts of the circuit.
The work done in moving a unit charge between two points of a circuit is called the terminal potential difference between those points.
Thus, if W joule of work has to be done by passing q coulomb of charge between two points of a valid circuit, then the terminal potential difference between those points will be V = W’ / q volt. The potential difference is measured with a voltmeter.
Internal Resistance of a Cell :
When we connect the plates of a cell by wire, then the valid current in the wire flows from the positive plate of the cell to the negative plate, and the solution inside the cell flows from the negative plate to the positive plate.
Just as the wire exerts resistance to the electric current, similarly the solution of the cell also imposes a resistance in the path of the electric current. This resistance is called the internal resistance of the cell.
Due to this resistance, some part of the energy given by the cell gets spent within the cell itself.
The internal resistance of the cell depends on the following factors.
- The distance between the two plates of the cell is directly proportional to.
- Is inversely proportional to the area of the dipped plates in solution.
- Increases with increasing concentration of electrolyte.
The internal resistance of the cell does not remain constant, but gradually increases as the cell is used.
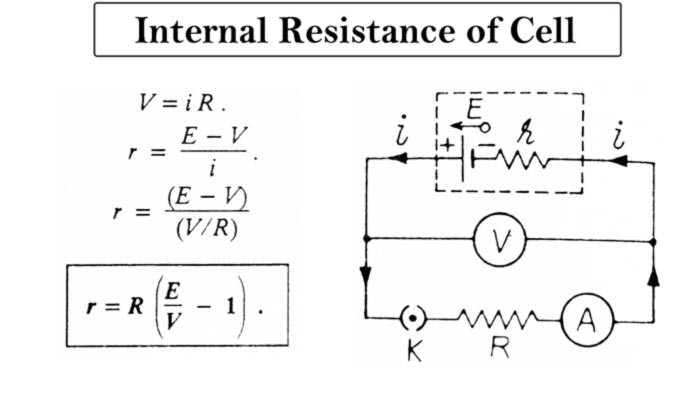
Terminal potential difference, EMF and internal resistance of the cell: A cell whose emf is E and internal resistance r is connected to a resistance wire R and aameter A by a key K. A voltmeter V is added between the plates of the cell.
The internal resistance of the cell can be understood to be added in series with the r cell as shown in the figure. On closing the key K, the cell starts sending a valid current to the circuit, whose value is read from the ammeter. Let this value be i and the value of voltmeter is V.
- Mole in chemistry: What is it and how to calculate it?
- What are the common uses of cesium in industry : Properties, Isotopes
- What is aluminum bromide and how is it used in industry?
- Exploring the Rankine Scale: Understanding the Basics and Applications
- What is Empirical Formula? How to Calculate Empirical Formula?
Let the current i in the circuit flow for t time. If t flows for time. If q is the charge flowing in the circuit in t time, then the energy given by the cell.
W = Eq
but q = it
so that W = E it
Outside the cell, the potential difference between the ends of the resistance R is V. Hence the work done outside the cell.
W = V it

The internal resistance of the cell is r. Therefore, when current i flows in the circuit, the potential drop in electrolyte inside the cell will be v = ir.
Hence the work done inside the cell
From the law of conservation of energy:
But V is also the terminal potential difference available between the plates of the cell. So it is clear that when the cell is giving current i.e. when it is discharging when the terminal potential difference V between its plates is less than its Emf E. The reason for this is the internal resistance in the cell, so that the potential drop inside the cell becomes ir.
It is clear from the above formula that the higher the value of i, the lesser the value of V will be. This means that the more current we take from the cell, the less will be the potential difference between its plates. thus
Each cell has its own characteristic and its value remains constant for a cell, whereas the value of terminal potential difference (V) decreases as more and more current is drawn from the cell.
If i = 0 in the above formula, then V = E
When current is not being drawn from the cell, then the terminal potential difference between the plates of the cell is equal to the emf of the cell. Therefore, when the key is open, the value of the voltmeter gives the value of Emf E of the cell.

This is the current i in the circuit, then the potential difference between the ends of the resistance R
V = iR
Here (R + r) is some (internal + external) resistance of the circuit. So it is clear that the value of the current drawn from the cell is obtained by the ratio of the emf of the cell and the total resistance of the circuit.
If there is more than one cell in the circuit, then the value of the current is obtained by the ratio of the net emf in the circuit and the total resistance of the circuit. This statement or the above formula expresses the law of conservation of energy for a valid circuit.
When current is drawn from the cell, the direction of current inside the cell is from the negative plate of the cell to the positive plate and the terminal potential difference V between the plates of the cell is less than the Emf E of the cell.
If we send current to the cell from any other valid source to charge a cell, then the direction of current inside the cell will be from positive plate to negative plate. In this case the terminal potential difference V between the plates of the cell will be more than the Emf E of the cell.
