The Gas Laws: Charle’s Law, Boyle’s Law and Gay-Lussac’s law|Chemistry Page

The Gas Laws: Charle’s Law, Boyle’s Law and Gay-Lussac’s law

Gas Laws

Matter is found in three physics states –

Solid: A solid is a substance whose shape and volume are fixed.

In the solid state of matter, the molecules of matter are very close to each other, that is, they have very little intermolecular space. Due to less intermolecular space, intermolecular attraction is very high in them and their freedom to move is very less.

In this way, their potential energy is very high and kinetic energy is very less and they keep on vibrating both in their mean position and in limited space. Due to this the size and volume of the solid remain fixed.

Liquid: A liquid is a substance whose volume remains fixed but the shape becomes like that of the vessel in which it is kept. The intermolecular space between the molecules of a liquid is greater than that of a solid. Due to this, the intermolecular attraction between the molecules of the liquid is less and the freedom of movement of the molecules of the liquid is more.

Thus the molecules of a liquid have less potential energy and more kinetic energy. Due to the high kinetic energy of liquid molecules, they keep on colliding with each other while moving randomly and they do not have any definite shape.

Because of this, the vessel in which the liquid is kept, therefore the liquid has the property of flowing and it does not have any definite shape. Due to this, the liquid assumes the shape of the vessel in which it is kept.

The kinetic energy of the liquid molecules is not more than the potential energy produced by their earth’s gravity and due to intermolecular attraction, so they cannot leave their plane and the volume of the liquid remains fixed.

Gas: A gas is a substance whose volume and shape are not fixed, but the volume and shape of the vessel in which it is kept becomes similar. The intermolecular space between gas molecules is much larger than that of solid and liquid.

Due to the large space between the gas molecules, the intermolecular attraction between them is very less and the freedom of movement of their molecules is very high. In this way, the potential energy of the gas molecules is very low and the kinetic energy is very high and they remain almost completely free.

Due to the high kinetic energy, the gas molecules collide with each other and with the walls of the vessel and move at an irregular speed in all directions. Because of this, gases also have the property of flowing like liquids and their shape is not fixed. The kinetic energy of gas molecules is so high that they can leave their plane. Therefore, the volume of gases is also uncertain.

Due to the high kinetic energy, the gas molecules
collide with each other and with the walls of the vessel and move at an
irregular speed in all directions. Because of this, gases also have the
property of flowing like liquids and their shape is not fixed. The kinetic
energy of gas molecules is so high that they can leave their plane. Therefore,
the volume of gases is also uncertain.

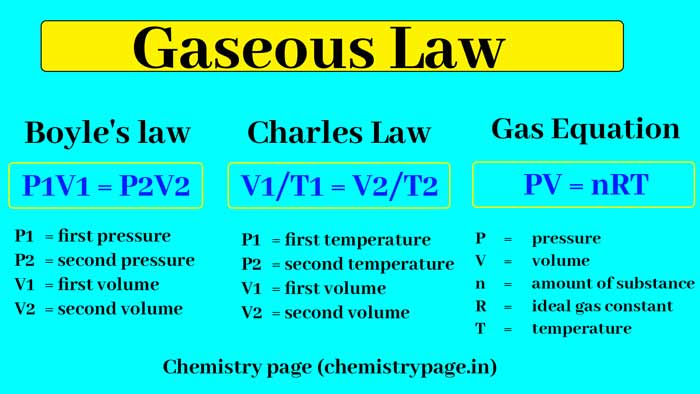
Boyle’s law

Boyle introduced this rule in 1662.

According to this rule :-

The volume (V) of a fixed amount of a gas at constant temperature is inversely proportional to its pressure (P).

V ∝ 1/P

V = k x 1/P (where k is a constant)

PV = k

Therefore, the product of pressure and volume of a gas at a constant temperature is always constant.

If a certain quantity of a gas at the same temperature has volume V1 and pressure P1 and its volume is changed from V1 to V2, then its pressure from P1 to P2 will be in such a way that –

P1V1 = P2V2

P1 = first pressure

P2 = second pressure

V1 = first volume

V2 = second volume

Charles Law

Charles introduced this rule in 1787. According to this law –

The volume of a fixed amount of a gas at constant pressure is directly proportional to its absolute temperature.

Absolute temperature = 273 + temperature in °C

The absolute temperature is represented in A° (°absolute) or K (kelvin). If the temperature of a gas is 25 °C, then its absolute temperature will be 273 + 25 = 298 K.

If the volume V and absolute temperature T of a certain
amount of a gas at constant pressure is –

V ∝ T

V = k’ T (k is a constant)

If a fixed volume of a gas at constant pressure has volume V1 and absolute temperature T1 and its temperature is raised from T1 to T2, then its volume from V1 to V2 will be such that –

V1/T1 = V2/T2

Putting the value of T1 as 273°A and the value of T2 as 274°A (1°C) –

V1/V2 = T1/T2 = 273/274

V1/V2 = 273/274 = 1 + 1/273

V2 = V1 + V1/273

Therefore, the volume of a certain amount of a gas at constant pressure increases by 1/273 of its volume on increasing its temperature from 0°C to 1°C. Similarly, on reducing the temperature from 0°C to -1°C, the volume of a gas decreases by 1/273 of its volume.

According to this law, the volume of gas will become 0 at absolute zero temperature. It is not possible to generate absolute temperature.

Gas Equation

By combining the laws of Boyle and Charles, these two laws can be represented by only one equation.

Boyle’s law V ∝ 1/P

Charles Law: V ∝ T

There for V ∝ T/P

PV ∝ T

PV = KT (K is a constant)

It is clear that both the above rules are included in the above equation. If T is constant then PV = KT = k, which is Boyles law. If P is constant then V = (KP) T = kT which is Charles law.

The value of K depends on the amount of gas and the units of P, V and T. According to Avogadro’s law, the volume of one gram molecule of all gases under the same conditions of temperature and pressure is also the same.

Therefore, if the equation PV = KT is used for one gram molecule of gases, then the value of K will remain the same for each gas. In this case K is replaced by R in the equation PV = KT and the equation PV = KT takes the following form –

PV = RT

Where

P = pressure of gas

V = Volume of one gram molecule of gas

R = gas constant or molecular gas constant

T = absolute temperature

The above equation is called gas equation. It can be used for one gram molecule of any gas. Its generalized form is as follows-

PV = nRT

Where

P = pressure of gas

V = volume of gas

R = gas constant or molecular gas constant

T = absolute temperature

n = number of moles of gas

= Amount of gas in grams / Molecular mass of gas

The above equation can also be written as –

PV = (w/M )RT

where w is the amount of gas in grams and M is the molecular weight of the gas.

If D is the density of the gas, then D = w / V

Therefore

p = D/M RT

According to the equation PV = nRT, if V and T are constant, then P ∝ n means that the pressure of a gas is proportional to the number of grams of its molecules.

Value of R

The value of R depends on the units of P , V and T. According to avogadro’s law, the volume of 1 gram molecule of all gases under conditions of normal temperature and pressure is 22.4 liter. Therefore, under normal temperature and pressure –

P = 1 atmospheric pressure

T = 273°A (0°C)

V = 22.4 liter

R = PV / T

= 1 x 22.4 / 273

= 0.0821 litre- atmospheric pressure per degree absolute temperature per gram atom

If P is represented in dine per squar cm and V in cub cm, then –

P = 1 atmospheric pressure

= 760 mm pressure of mercury

= 76 cm pressure of mercury

= 76 x 13.6 x 981 dine per squar cm

V = 22.4 liter

= 22400 cube cm

T = 273°A (0°C)

R = PV / T = (76 x 13.6 x 981 x 22400) / 273

= 8.3 x 107 arg per degree absolute temperature per gram atom

= 8.3 Joule per degree absolute temperature per gram atom

We know that 1 calory of energy is equal to 4.18 x 107 arg work.

Therefore

R = 8.3 x 107 / 4.18 x 107

≈ 2 Calories per degree absolute temperature per gram atom

Uses of Gas Equation

In chemical reactions in which gases take part as reactants or are obtained as a product, there is great difficulty in finding out by weighting the quantities of gases used or obtained from them. In these cases, the gas equation is used to find the quantity of gases.

First the volume of the gas is determined and then this volume is converted by the gas equation at N.T.P.. If the volume of the gas at P1 pressure and T1 temperature is V1, then the volume V2 of the gas at P2 pressure and T2 temperature can be found as follows –

P1V1 = nRT1

P2V2 = nRT2

P1V1/T1 = P2V2/ T2

To change the volume of gas at N.T.P., the value of P2 is an atmospheric pressure (76 cm of mercury) and the value of T2 is 273°A. With the help of avogadro’s law from the volume thus determined, the mass of the gas can be found. According to avogadro’s law the mass of 22.4 liters of each gas at N.T.P. is equal to its gram molecular mass.

Gay-Lussac’s law of gaseous volumes

Gay Lussac studied the behavior of gases in 1798 and on this basis formulated the rule regarding gaseous volumes.

According to this rule –

When gases chemically combine with each other, their volumes are in a simple ratio at the same temperature and pressure and if the substances formed by their combination are also in gaseous form, then at the same temperature and pressure, their volumes are also in the same ratio. There is a simple ratio.

Avogadro’s Law

BERZELIUS tried to explain Gay-Lussac’s law of gaseous volume on the basis of Dalton’s atomic. For this berzelius presented a hypothesis. Avogadro (1811) revised this hypothesis. The truth of avogadro’s hypothesis has been confirmed by experiments and it has taken the form of a rule.

According to avogadro’s law –

The same volume of gases at the same temperature and pressure have the same number of molecules.

Avogadro’s law has made an important contribution in the development of chemistry, with its help the following important conclusions are obtained –

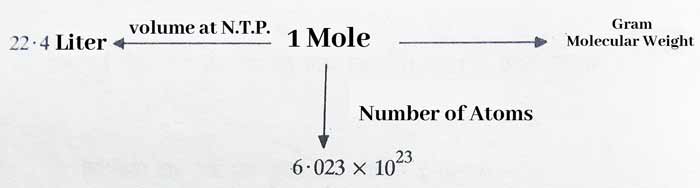
1. The mass of 22.4 liters of a gas at 1 N.T.P. is equal to its gram molecular mass.

1 mole of a gas is equal to its gram molecular weight and 1 mole of a gas contains 6.023 x 1023 molecules. The above facts can also be written together as follows –

mole

Molecular Weight = 2 x Vapor Density

The vapor density of a gas is equal to the ratio of its density to that of hydrogen under the same conditions of temperature and pressure.

Vapor Density = Density of gas / Density of H2 at same Temperature and pressure

= molar mass of gas / molar mass of H2

The mass of 22.4 liters of a gas at N.T.P. is equal to its gram molecular mass. The weight of 22.4 liters of hydrogen at N.T.P. is 2 grams.

Molecular Weight = 2 Vapor Density

Therefore, there is no unit of vapor density. Its value depends on temperature and pressure. The vapor density of hydrogen is 1. The density of a substance is the amount of its unit volume. If the density of the substance is D, the volume is V and the mass is M, then –

D = M / V

Its units are garm / ml , gram / l , gram / cub cm etc. The expected density of a substance is equal to the numerical value of its density in grams/ml. The vapor density of a gas is 11200 times greater than its density in grams/ml at N.T.P..

At the same temperature and pressure –

Vapor Density ∝ Density

Vapor Density of Gas A/Vapor Density of Gas B = Density of Gas A/Density of Gas B

The vapor density of a gas mixture can be determined by definition with the help of experiments. The vapor density of a gaseous mixture can also be found by dividing its average molecular mass by 2.

Molecular Weight(Gas Mixture) = Total weight of Gas Mixture / Number of Moles(Present atoms of gas mixture)

3. In gaseous reactions, the ratio of the volumes of reactants and products is the same as that of their molecules in the chemical equation of the reaction. Its converse is also true.

Molar volume:-

The volume of one gram molecule (one mole) of a gas is called its gram molecular volume. The gram molecular volume of each gas at N.T.P. is 22.4 liters. The molecular weight of oxygen is 32, so the volume of 32 grams of oxygen at N.T.P. is 22.4 liters.

Stay Connected

Editor's Choice

Introduction of Inductive-Effect || How does Inductive Effect Work?

2023-09-19